The main research aim of our group is the development and analysis of individual-based mathematical models of collective behavior, which explicitly take the sensory perception at the individual level into account, as the proximate mechanism of social interactions in organismic systems (insects, fish, birds). Using our modeling approach, which combines state-of-art statistical physics methods, simplified models of sensory perception, and direct empirical input from our experimental partners, we aim at a systematic investigation of fundamental properties and limitations of sensory perception in the context of information transfer within animal groups. Our theoretical work focuses on modeling, mathematical analysis, and numerical simulations. However, we collaborate closely with the Couzin Lab at the Department of Collective Behavior, MPI for Ornithology / University Konstanz, the Krause Lab, Department of Fish Biology and Ecology at the IGB Berlin, and the Berlin Biorobotics Lab headed by Tim Landgraf .
Our main research activity focuses on the following interconnected research questions:
- Do schools, flocks & crowds – from cellular agregates to animal groups (incl. humans) – share common functional features as collective information processing system?
- How does collective behavior emerge from sensory inputs? Here, we focus on question on how individuals integrate visual social information and use it to coordinate their actions.
- How do animal groups perform collective decisions under sensory constraints? Here, the central question is how animal groups are able to perform near-optimal and robust collective decisions despite sensory constraints and highly variable environments.
- What is the interplay between the self-organized structure and dynamics of collective systems and their collectively intelligent (or not so intelligent) behavior?
Ongoing projects:
- Modeling of collective decision processes in time and space (with R. Kurvers, H. Hamann & others)
- Modeling of behavioral contagion in a controlled laboratory setting (together with the Couzin Lab and Bryan Daniels, Colin Twomey, Winnie Poel)
- Observation, quantification and modeling of large scale collective cascades in fish in the wild (together with Jens Krause, David Bierbach, Juliane Lukas, Pascal Klamser and others)
- Large scale coordination and flocking in heterogeneous environments (with Parisa Rahmani and Fernando Peruani) and in different flocking models (with Cristian Huepe and Yinong Zhao)
- Coordination in multicellular animals (with Mircea Davidescu, Iain Couzin and Thomas Gregor)
Further research interest (current & past research):
Emergence of Collective Motion and Novel States of Active Matter
Onset of collective behavior in models models with empirically motivated, phenomological interactions, which go beyond ‘minimal’ alignment models, e.g. selective attraction-repulsion or combined alignment+anti-alignment interactions; Emergence of novel states of active matter in simple self-propelled particle systems, different from the orientationally ordered fluid states (Toner and Tu), such as active turbulence, or spatially periodic structures (active smectic pattern, active crystals).
See e.g.
- Großmann et al. (2014). Vortex Arrays and Mesoscale Turbulence of Self-Propelled Particles. Phys Rev Lett, 113(25), 258104.
- Großmann et al. (2015). Pattern formation in active particle systems due to competing alignment interactions. Eur Phys J Special Topics, 224(7), 1325–1347.
- Romanczuk et al. (2016). Emergent smectic order in simple active particle models, New Journal of Physics 18(6), 063015 (2016)
Impact of spatio-temporal behavioral constraints on cooperation and competition
Understanding of evolutionary constraints and biological function by simple evolutionary models directly motivated by experimental observation, which include empirically observed spatio-temporal constraints.
see e.g.
- Monk et al. (2018) How ecology shapes exploitation: a framework to predict the behavioural response of human and animal foragers along exploration–exploitation trade‐offs , Ecology Letters, 21, 6
- Guttal et al. (2012). Cannibalism can drive the evolution of behavioural phase polyphenism in locusts. Ecology Letters, 15(10), 1158–1166.
- Herbert-Read & Romanczuk et al., (2016). Proto-cooperation: Group hunting sailfish improve hunting success by alternating attacks on grouping prey, Proc. R. Soc. B, 283(1842), 20161671 (2016) preprint
Coarse-grained mathematical description of active matter systems
 Derivation of coarse-grained (hydrodynamic) equation of motion for macroscopic observables such as density or momentum fields from microscopic equations of motion for interacting self-propelled particle systems. Systematic analysis of the resulting hydrodynamic equations, which enable us to construct phase diagrams for the corresponding model systems.
Derivation of coarse-grained (hydrodynamic) equation of motion for macroscopic observables such as density or momentum fields from microscopic equations of motion for interacting self-propelled particle systems. Systematic analysis of the resulting hydrodynamic equations, which enable us to construct phase diagrams for the corresponding model systems.
See e.g.
- Romanczuk & Schimansky-Geier (2012). Mean-field theory of collective motion due to velocity alignment. Ecol. Complexity, 10, 83–92.
- Großmann et al (2013). Self-propelled particles with selective attraction–repulsion interaction: from microscopic dynamics to coarse-grained theories. New J Phys, 15(8), 085014.
- Großmann et al. (2014). Vortex Arrays and Mesoscale Turbulence of Self-Propelled Particles. Phys Rev Lett, 113(25), 258104.
Modeling Individual and Collective Chemotaxis
Agent-based stochastic models of individual directed migration and collective behavior mediated by chemoattractants.
see e.g.
- P Romanczuk, U Erdmann, H Engel, L Schimansky-Geier (2008), Beyond the Keller-Segel model Microscopic modelling of bacterial colonies, Eur Phys J-Spec Top, 157:61-77
- M. Meyer, L. Schimansky-Geier, P. Romanczuk (2014), Active Brownian particles with concentration-dependent chemotactic sensitivity, Phys Rev E 89, 022711
- A. Diz-Munoz, P. Romanczuk, W. Yu, M. Bergert, P. Dieterich, G. Salbreux, C.-P. Heisenberg, E. Paluch, Complementary functions of blebs and lamellipodia in steering cell migration in vivo, BMC Biology 14, 74 (2016)
Stochastic dynamics far from equilibrium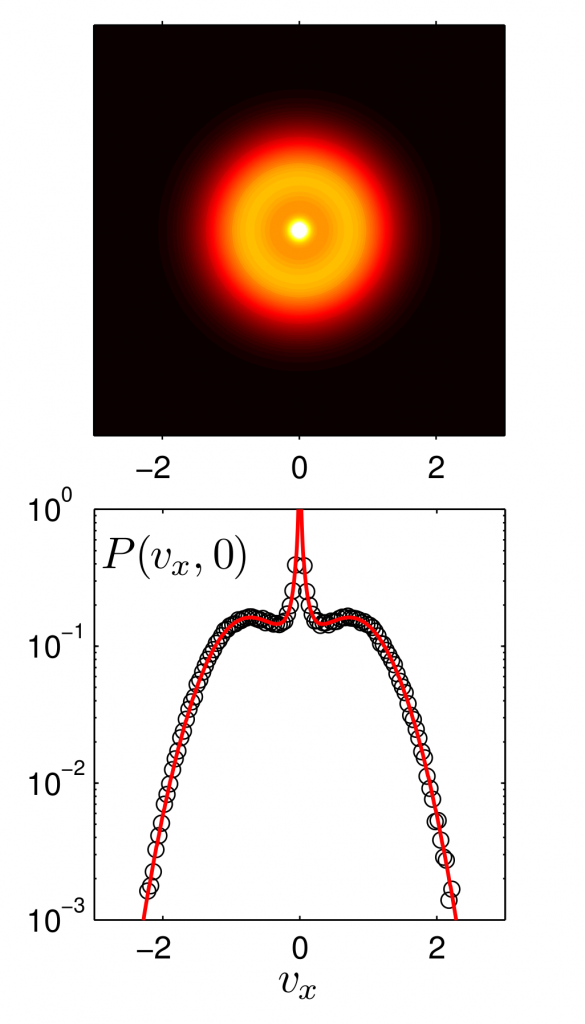
Modeling of physical and biological systems using stochastic differential equation, with a focus on the theory and dynamics of so-called active Brownian particles.
see e.g.
- Romanczuk & Schimansky-Geier. (2011). Brownian Motion with Active Fluctuations. Phys Rev Lett, 106(23), 230601.
- Romanczuk et al. (2012). Active Brownian particles. The European Physical Journal – Special Topics, 202(1), 1–162.
